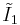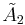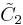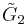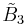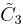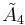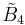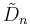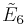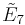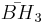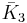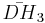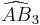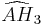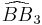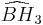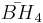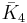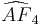Coxeter notation

In geometry, Coxeter notation is a system of classifying symmetry groups, describing the angles between with fundamental reflections of a Coxeter group. It uses a bracketed notation, with modifiers to indicate certain subgroups. The notation is named after H. S. M. Coxeter, and has been more comprehensively defined by Norman Johnson.

Contents

Reflectional groups

Further information: Point group

For Coxeter groups defined by pure reflections, there is a direct correspondence between the bracket notation and Coxeter-Dynkin diagram graphs. The numbers in the bracket notation represent the mirror reflection orders in the branches of the Coxeter graph.

The Coxeter notation is simplified with exponents to represent the number of branches in a row for linear graphs. So the An group is represented by [3n-1], to imply n nodes connected by n-1 order-3 branches.

Further branching graphs began as numbers given with vertical positions in the brackets, but simplified into multiple superscript values at the branch lengths.

Coxeter groups formed by cyclic graphs are represented by parenthesese inside of brackets, like [(a,b,c)] for the triangle group (a b c). If they are equal, they can be grouped as an exponent as the length the cycle in brackets, like [(3,3,3,3)] = [3[4]].

Finite Coxeter groups
Rank Group
symbol		 Bracket notation Coxeter graph
2 A2 [3]
2 BC2 [4]
2 H2 [5]
2 G2 [6]
2 I2(p) [p]
3 H3 [5,3]
3 A3 [3,3]
3 BC3 [4,3]
4 A4 [3,3,3]
4 BC4 [4,3,3]
4 D4 [31,1,1]
4 F4 [3,4,3]
4 H4 [5,3,3]
n An [3n-1] ..
n BCn [4,3n-2] ...
n Dn [3n-3,1,1] ...
6 E6 [32,2,1]
7 E7 [33,2,1]
8 E8 [34,2,1]
Affine Coxeter groups
Group
symbol		 Bracket notation Coxeter graph
{\tilde{I}}_1 [∞]
{\tilde{A}}_2 [3[3]]
{\tilde{C}}_2 [4,4]
{\tilde{G}}_2 [6,3]
{\tilde{A}}_3 [3[4]]
{\tilde{B}}_3 [4,31,1]
{\tilde{C}}_3 [4,3,4]
{\tilde{A}}_4 [3[5]]
{\tilde{B}}_4 [4,3,31,1]
{\tilde{C}}_4 [4,3,3,4]
{\tilde{D}}_4 [ 31,1,1,1]
{\tilde{F}}_4 [3,4,3,3]
{\tilde{A}}_n [3[n+1]] ...
or
...
{\tilde{B}}_n [4,3n-2,31,1] ...
{\tilde{C}}_n [4,3n-1,4] ...
{\tilde{D}}_n [ 31,1,3n-3,31,1] ...
{\tilde{E}}_6 [32,2,2]
{\tilde{E}}_7 [33,3,1]
{\tilde{E}}_8 [35,2,1]
Compact Hyperbolic Coxeter groups
Group
symbol		 Bracket notation Coxeter graph
[p,q]
with 2(p+q)<pq
[(p,q,r)]
with p+q+r>9
{\bar{BH}}_3 [4,3,5]
{\bar{K}}_3 [5,3,5]
{\bar{J}}_3 [3,5,3]
{\bar{DH}}_3 [5,31,1]
{\widehat{AB}}_3 [(3,3,3,4)]  
{\widehat{AH}}_3 [(3,3,3,5)]  
{\widehat{BB}}_3 [(3,4,3,4)]
{\widehat{BH}}_3 [(3,4,3,5)]
{\widehat{HH}}_3 [(3,5,3,5)]
{\bar{H}}_4 [3,3,3,5]
{\bar{BH}}_4 [4,3,3,5]
{\bar{K}}_4 [5,3,3,5]
{\bar{DH}}_4 [5,3,31,1]
{\widehat{AF}}_4 [(3,3,3,3,4)]

For the affine and hyperbolic groups, the subscript is one less than the number of nodes in each case, since each of these groups was obtained by adding a node to a finite group's graph.

The Coxeter graph usually leaves order-2 branches undrawn, but the bracket notation includes an explicit 2 to connect the subgraphs. So the Coxeter graph, , H3×A2 can be represented by [5,3]×[3] and [5,3,2,3].

By rank

Coxeter groups are categorized by their rank, being the number of nodes in its Coxeter graph. The structure of the groups are also given with their abstract group types: In this article, the abstract dihedral groups are represented as Dihn, and cyclic groups are represented by Zn, with Dih1=Z2.

Rank one groups

In one dimension, the bilateral group [ ] represents a single mirror symmetry, abstract Dih1 or Z2, symmetry order 2. It is represented as a Coxeter graph with a single node, . The identity group is the direct subgroup [ ]+, Z1, symmetry order 1. The + superscript simply implies that alternate mirror reflections are ignored, leaving the identity group in this simplest case.

Group Coxeter Coxeter diagram Order Description
C1 [ ]+ 1 Identity
D1 [ ] 2 Reflection group

Rank two groups

In two dimensions, the rectangular group [2], abstract Dih2, also can be represented as a direct product [ ]×[ ] or Z2×Z2, being the product of two bilateral groups, represents two orthogonal mirrors, with Coxeter graph, , with order 4. The 2 in [2] comes from linearization of the orthogonal subgraphs in the Coxeter graph, as , with explicit branch order 2. The rhombic group, [2]+, half of the rectangular group, the point reflection symmetry, Z2, order 2.

Coxeter notation allows a 1 place-holder for lower rank groups, so [1] is the same as [ ], and [1]+ is the same as [ ]+. This may be done to imply the group exists in 2-dimensions rather than 1 dimension.

Coxeter notation uses double-bracking to represent an automorphic doubling of symmetry by adding a bisecting mirror to the fundamental domain. For example [[p]] adds a bisecting mirror to [p], and is isomorphic to [2p].

The full p-gonal group [p], abstract dihedral group Dihp, (nonabelian for p>2), of order 2p, is generated by two mirrors at angle π/p, represented by Coxeter graph . The p-gonal subgroup [p]+, cyclic group Zp, of order p, generated by a rotation angle of π/p.

In the limit, going down to one dimensions, the full apeirogonal group is obtained when the angle goes to zero, so [∞], abstractly the infinite dihedral group Dih∞, represents two parallel mirrors and has a Coxeter graph . The apeirogonal group [∞]+, abstractly the infinite cyclic group Z∞, isomorphic to the additive group of the integers, is generated by a single nonzero translation.

In the hyperbolic plane, there's a full pseudogonal group [πi/λ], and pseudogonal subgroup [πi/λ]+. These groups exist in regular infinite sided polygons, with edge length λ. The mirrors are all orthogonal to a single line.

Group Intl Orbifold Coxeter Order Description
Finite
Zn n nn [n]+ n Cyclic: n-fold rotations. Abstract group Zn, the group of integers under addition modulo n.
Dn nm *nn [n] 2n Dihedral: cyclic with reflections. Abstract group Dihn, the dihedral group.
Affine
Z∞ ∞ ∞∞ [∞]+ ∞ Cyclic: apeirogonal group. Abstract group Z∞, the group of integers under addition.
Dih∞ ∞m *∞∞ [∞] ∞ Dihedral: parallel reflections. Abstract infinite dihedral group Dih∞.
Hyperbolic
Z∞ [πi/λ]+ ∞ pseudogonal group
Dih∞ [πi/λ] ∞ full pseudogonal group

Rank three groups

Further information: List of spherical symmetry groups‎ and List of planar symmetry groups

In three dimensions, the full orthorhombic group [2,2], astracttly Z2×Dih2, order 8, represents three orthogonal mirrors, and also can be represented by Coxeter graph as three separate dots . It can also can be represented as a direct product [ ]×[ ]×[ ], but the [2,2] expression allows subgroups to be defined:

First there is a semidirect subgroup, the orthorhombic group, [2,2+], abstractly Dih1×Z2=Z2×Z2, of order 4. When the + superscript is given inside of the brackets, it means reflections generated only from the adjacent mirrors (as defined by the Coxeter graph, ) are alternated. In general, the branch orders neighboring the + node must be even. In this case [2,2+] and [2+,2] represent two isomorphic subgroups that are geometrically distinct. The other subgroups are the pararhombic group [2,2]+, also order 4, and finally the central group [2+,2+] of order 2.

Next there is the full ortho-p-gonal group, [2,p], abstractly Dih1×Dihp=Z2×Dihp, of order 4p, representing two mirrors at a dihedral angle π/p, and both are orthogonal to a third mirror. It is also represented by Coxeter graph as .

The direct subgroup is called the para-p-gonal group, [2,p]+, abstractly Dihp, of order 2p, and another subgroup is [2,p+] abstractly Zp×Z2, also of order 2p.

The full gyro-p-gonal group, [2+,2p], abstractly Dih2p, of order 4p. The gyro-p-gonal group, [2+,2p+], abstractly Z2p, of order 2p is a subgroup of both [2+,2p] and [2,2p+].

The polyhedral groups are based on the symmetry of platonic solids, the tetrahedron, octahedron, cube, icosahedron, and dodecahedron, with Schläfli symbols {3,3}, {3,4}, {4,3}, {3,5}, and {5,3} respectively. The Coxeter groups for these are called in Coxeter's bracket notation [3,3], [3,4], [3,5] called full tetrahedral symmetry, octahedral symmetry, and icosahedral symmetry, with orders of 24, 48, and 120. The order of the number in the Coxeter notation don't make a difference, unlike the Schläfli symbol.

The tetrahedral group, [3,3], has a doubling [[3,3]] which maps the first and last mirrors onto each other, and this produces the [3,4] group.

In all these symmetries, alternate reflections can be removed producing the rotational tetrahedral, octahedral, and icosahedral groups of order 12, 24, and 60. The octahedral group also has a unique subgroup called the pyritohedral symmetry group, [3+,4], of order 12, with a mixture of rotational and reflectional symmetry.

In the Euclidean plane there's 3 fundamental reflective groups generated by 3 mirrors, represented by Coeter graphs , , and , and are given Coxeter notation as [4,4], [6,3], and [(3,3,3)]. The parentheses of the last group imply the graph ic cyclic, and also has a short-hand notation [3[3]].

[[4,4]] as a doubling of the [4,4] group produced the same symmetry rotated π/4 from the original set of mirrors.

Direct subgroups of rotational symmetry are: [4,4]+, [6,3]+, and [(3,3,3)]+. [4+,4] and [6,3+] represent mixed reflectional and rotational symmetry.

Finite
Intl* Geo
[1]		 Orbifold Schönflies Conway Coxeter Order
1 1 1 C1 C1 [ ]+ 1
1 22 ×1 Ci = S2 CC2 [2+,2+] 2
2 = m 1 *1 Cs = C1v = C1h ±C1 = CD2 [ ] 2
2
3
4
5
6
n		 2
3
4
5
6
n		 22
33
44
55
66
nn		 C2
C3
C4
C5
C6
Cn		 C2
C3
C4
C5
C6
Cn		 [2]+
[3]+
[4]+
[5]+
[6]+
[n]+
 2
3
4
5
6
n
2mm
3m
4mm
5m
6mm
nmm
nm		 2
3
4
5
6
n		 *22
*33
*44
*55
*66
*nn		 C2v
C3v
C4v
C5v
C6v
Cnv		 CD4
CD6
CD8
CD10
CD12
CD2n		 [2]
[3]
[4]
[5]
[6]
[n]		 4
6
8
10
12
2n
2/m
3/m
4/m
5/m
6/m
n/m		 2 2
3 2
4 2
5 2
6 2
n 2		 2*
3*
4*
5*
6*
n*		 C2h
C3h
C4h
C5h
C6h
Cnh		 ±C2
CC6
±C4
CC10
±C6
±Cn / CC2n		 [2,2+]
[2,3+]
[2,4+]
[2,5+]
[2,6+]
[2,n+]		 4
6
8
10
12
2n
4
3
8
5
12
2n
n		 4 2
6 2
8 2
10 2
12 2
2n 2		 2×
3×
4×
5×
6×
n×		 S4
S6
S8
S10
S12
S2n		 CC4
±C3
CC8
±C5
CC12
CC2n / ±Cn		 [2+,4+]
[2+,6+]
[2+,8+]
[2+,10+]
[2+,12+]
[2+,2n+]		 4
6
8
10
12
2n
Intl Geo Orbifold Schönflies Conway Coxeter Order
222
32
422
52
622
n22
n2		 2 2
3 2
4 2
5 2
6 2
n 2		 222
223
224
225
226
22n		 D2
D3
D4
D5
D6
Dn		 D4
D6
D8
D10
D12
D2n		 [2,2]+
[2,3]+
[2,4]+
[2,5]+
[2,6]+
[2,n]+		 4
6
8
10
12
2n
mmm
6m2
4/mmm
10m2
6/mmm
n/mmm
2nm2		 2 2
3 2
4 2
5 2
6 2
n 2		 *222
*223
*224
*225
*226
*22n		 D2h
D3h
D4h
D5h
D6h
Dnh		 ±D4
DD12
±D8
DD20
±D12
±D2n / DD4n		 [2,2]
[2,3]
[2,4]
[2,5]
[2,6]
[2,n]		 8
12
16
20
24
4n
42m
3m
82m
5m
122m
2n2m
nm		 4 2
6 2
8 2
10 2
12 2
n 2
 2*2
2*3
2*4
2*5
2*6
2*n		 D2d
D3d
D4d
D5d
D6d
Dnd		 ±D4
±D6
DD16
±D10
DD24
DD4n / ±D2n		 [2+,4]
[2+,6]
[2+,8]
[2+,10]
[2+,12]
[2+,2n]		 8
12
16
20
24
4n
23 3 3 332 T T [3,3]+ 12
m3 4 3 3*2 Th ±T [3+,4] 24
43m 3 3 *332 Td TO [3,3] 24
432 4 3 432 O O [3,4]+ 24
m3m 4 3 *432 Oh ±O [3,4] 48
532 5 3 532 I I [3,5]+ 60
53m 5 3 *532 Ih ±I [3,5] 120
Semiaffine
Intl (orbifold) Geo
 Schönflies Coxeter Order
n nn n Cn [1,n]+ n
nm *nn n Dn [1,n] 2n
IUC (Orbifold) Geo Schönflies Coxeter
p1 ∞∞ p1 C∞ [1,∞]+
p1m1 *∞∞ p1 C∞v [1,∞]
IUC (Orbifold) Geo Schönflies Coxeter
p11g ∞x p.g1 S2∞ [∞+,2+]
p11m ∞* p.1 C∞h [∞+,2]
p2 22∞ p2 D∞ [∞,2]+
p2mg 2*∞ p2g D∞d [∞,2+]
p2mm *22∞ p2 D∞h [∞,2]
Affine
IUC (Orbifold) Geometric Coxeter
p2 (2222) p2 [1+,4,4]+
p2gg (22x) pg2g [4+,4+]
p2mm (*2222) p2 [1+,4,4]
c2mm (2*22) c2 [[4+,4+]]
p4 (442) p4 [4,4]+
p4gm (4*2) pg4 [4+,4]
p4mm (*442) p4 [4,4]
IUC (Orbifold) Geometric Coxeter
p3 (333) p3 [1+,6,3+] = [3[3]]+
p3m1 (*333) p3 [1+,6,3] = [3[3]]
p31m (3*3) h3 [6,3+] = [3[3[3]]+]
p6 (632) p6 [6,3]+ = [3[3[3]]]+
p6mm (*632) p6 [6,3] = [3[3[3]]]

Rank four groups

Point groups

Rank four groups defined the 4-dimensional point groups:

Finite groups
[ ]:
Symbol Order
[1]+ 1.1
[1] = [ ] 2.1
[2,1,1]:
Symbol Order
[2,1,1]+ 2.1
[2,1,1] 4.1
[2,2,1]:
Symbol Order
[2+,2+,1] 2.1
[2,2,1]+ 4.1
[2+,2,1] 4.1
[2,2,1] 8.1
[2,2,2]:
Symbol Order
[2+,2+,2+] 2.1
[2+,2,2+] 4.1
[(2,2)+,2+] 4
[[2+,2+,2+]] 4
[2,2,2]+ 8
[2+,2,2] 8.1
[(2,2)+,2] 8
[[2+,2,2+]] 8.1
[2,2,2] 16.1
[[2,2,2]]+ 16
[[2,2+,2]] 16
[[2,2,2]] 32
[p,1,1]:
Symbol Order
[3,1,1]+ 3.1
[4,1,1]+ 4.2
[5,1,1]+ 5.1
[6,1,1]+ 6.1
[p,1,1]+ p
[3,1,1] 6.2
[4,1,1] 8.4
[5,1,1] 10.2
[6,1,1] 12.3
[p,1,1] 2p
[p,2,1]:
Symbol Order
[3,2,1]+ 6.1
[4,2,1]+ 8.3
[5,2,1]+ 10.2
[6,2,1]+ 12.3
[p,2,1]+ 2p
[3,2,1] 12.3
[4,2,1] 16.6
[5,2,1] 20.3
[6,2,1] 24.6
[p,2,1] 4p
[2p,2+,1]:
Symbol Order
[6,2+,1] 12.3
[8,2+,1] 16.12
[10,2+,1] 20.3
[12,2+,1] 24.12
[2p,2+,1] 4p
[p,2,2]:
Symbol Order
[3+,2,2+] 6
[4+,2,2+] 8
[5+,2,2+] 10
[6+,2,2+] 12
[p+,2,2+] 2p
[(3,2)+,2+] 6
[(4,2)+,2+] 8
[(5,2)+,2+] 10
[(6,2)+,2+] 12
[(p,2)+,2+] 2p
[3,2,2]+ 12
[4,2,2]+ 16
[5,2,2]+ 20
[6,2,2]+ 24
[p,2,2]+ 4p
[3,2,2+] 12
[4,2,2+] 16
[5,2,2+] 20
[6,2,2+] 24
[p,2,2+] 4p
[3+,2,2] 12
[4+,2,2] 16
[5+,2,2] 20
[6+,2,2] 24
[p+,2,2] 4p
[(3,2)+,2] 12
[(4,2)+,2] 16
[(5,2)+,2] 20
[(6,2)+,2] 24
[(p,2)+,2] 4p
[3,2,2] 24
[4,2,2] 32
[5,2,2] 40
[6,2,2] 48
[p,2,2] 8p
[2p,2+,2]:
Symbol Order
[6+,2+,2+] 6
[8+,2+,2+] 8
[10+,2+,2+] 10
[12+,2+,2+] 12
[2p+,2+,2+] 2p
[6+,2+,2] 12
[8+,2+,2] 16
[10+,2+,2] 20
[12+,2+,2] 24
[2p+,2+,2] 4p
[6+,(2,2)+] 12
[8+,(2,2)+] 16
[10+,(2,2)+] 20
[12+,(2,2)+] 24
[2p+,(2,2)+] 4p
[6,(2,2)+] 24
[8,(2,2)+] 32
[10,(2,2)+] 40
[12,(2,2)+] 48
[2p,(2,2)+] 8p
[6,2+,2] 24
[8,2+,2] 32
[10,2+,2] 40
[12,2+,2] 48
[2p,2+,2] 8p
[p,2,q]:
Symbol Order
[3+,2,3+] 9
[4+,2,3+] 12
[5+,2,3+] 15
[6+,2,3+] 18
[4+,2,4+] 16
[5+,2,4+] 20
[6+,2,4+] 24
[5+,2,5+] 25
[6+,2,5+] 30
[6+,2,6+] 36
[p+,2,q+] pq
[3,2,3]+ 18
[4,2,3]+ 24
[5,2,3]+ 30
[6,2,3]+ 36
[4,2,4]+ 32
[5,2,4]+ 40
[6,2,4]+ 48
[5,2,5]+ 50
[6,2,5]+ 60
[6,2,6]+ 72
[p,2,q]+ 2pq
[3+,2,3] 18
[4+,2,3] 24
[5+,2,3] 30
[6+,2,3] 36
[4+,2,4] 32
[5+,2,4] 40
[6+,2,4] 48
[5+,2,5] 50
[6+,2,5] 60
[6+,2,6] 72
[p+,2,q] 2pq
[3,2,3] 36
[4,2,3] 48
[5,2,3] 60
[6,2,3] 72
[4,2,4] 64
[5,2,4] 80
[6,2,4] 96
[5,2,5] 100
[6,2,5] 120
[6,2,6] 144
[p,2,q] 4pq
[(p,2)+,2q]:
Symbol Order
[(3,2)+,6+] 18
[(4,2)+,6+] 24
[(5,2)+,6+] 30
[(6,2)+,6+] 36
[(4,2)+,8+] 32
[(5,2)+,8+] 40
[(6,2)+,8+] 48
[(5,2)+,10+] 50
[(6,2)+,10+] 60
[(6,2)+,12+] 72
[(p,2)+,2q+] 2pq
[(3,2)+,6] 36
[(4,2)+,6] 48
[(5,2)+,6] 60
[(6,2)+,6] 72
[(4,2)+,8] 64
[(5,2)+,8] 80
[(6,2)+,8] 96
[(5,2)+,10] 100
[(6,2)+,10] 120
[(6,2)+,12] 144
[(p,2)+,2q] 4pq
[2p,2+,2q]:
Symbol Order
[6+,2+,6+] 18
[8+,2+,6+] 24
[10+,2+,6+] 30
[12+,2+,6+] 36
[8+,2+,8+] 32
[10+,2+,8+] 40
[12+,2+,8+] 48
[10+,2+,10+] 50
[12+,2+,10+] 60
[12+,2+,12+] 72
[2p+,2+,2q+] 2pq
[6,2+,6+] 36
[8,2+,6+] 48
[10,2+,6+] 60
[12,2+,6+] 72
[8,2+,8+] 64
[10,2+,8+] 80
[12,2+,8+] 96
[10,2+,10+] 100
[12,2+,10+] 120
[12,2+,12+] 144
[2p,2+,2q+] 4pq
[6,2+,6] 72
[8,2+,6] 96
[10,2+,6] 120
[12,2+,6] 144
[8,2+,8] 128
[10,2+,8] 160
[12,2+,8] 192
[10,2+,10] 200
[12,2+,10] 240
[12,2+,12] 288
[2p,2+,2q] 8pq
[[p,2,p]]:
Symbol Order
[[3+,2,3+]] 18
[[4+,2,4+]] 32
[[5+,2,5+]] 50
[[6+,2,6+]] 72
[[p+,2,p+]] 2p2
[[3,2,3]]+ 36
[[4,2,4]]+ 64
[[5,2,5]]+ 100
[[6,2,6]]+ 144
[[p,2,p]]+ 4p2
[[3,2,3]] 72
[[4,2,4]] 128
[[5,2,5]] 200
[[6,2,6]] 288
[[p,2,p]] 8p2
[[2p,2+,2p]]:
Symbol Order
[[6+,2+,6+]] 36
[[8+,2+,8+]] 64
[[10+,2+,10+]] 100
[[12+,2+,12+]] 144
[[2p+,2+,2p+]] 4p2
[[6,2+,6]] 144
[[8,2+,8]] 256
[[10,2+,10]] 400
[[12,2+,12]] 576
[[2p,2+,2p]] 16p2
[3,3,1]:
Symbol Order
[3,3,1]+ 12.5
[3,3,1] 24
[4,3,1]:
Symbol Order
[4,3,1]+ 24.15
[3+,4,1] 24.10
[4,3,1] 48.36
[5,3,1]:
Symbol Order
[5,3,1]+ 60.13
[5,3,1] 120.2
[3,3,2]:
Symbol Order
[(3,3)+,2] 24
[3,3,2]+ 24
[3,3,2] 48
[4,3,2]:
Symbol Order
[(4,3)+,2+] 24
[4,(3,2)+] 48
[(4,3)+,2] 48
[4,3,2]+ 48
[4,3+,2] 48.22
[4,3,2] 96.5
[5,3,2]:
Symbol Order
[(5,3)+,2] 120.2
[5,3,2]+ 120.2
[5,3,2] 240
[31,1,1]:
Symbol Order
[31,1,1]+
= [1+,4,3,3]+		 96.1
[31,1,1]
= [1+,4,3,3]		 192
<[3,31,1]>
= [4,3,3]		 384.1
[3[31,1,1]]
= [3,4,3]		 1152.1
[3,3,3]:
Symbol Order
[3,3,3]+ 60
[3,3,3] 120.1
[[3,3,3]]+ 120.2
[[3,3,3]+] 120.1
[[3,3,3]] 240.1
[4,3,3]:
Symbol Order
[1+,4,3,3]+
= [3,31,1]+		 96.1
[1+,4,3,3]
= [3,31,1]		 192
[4,(3,3)+] 192
[4,3,3]+ 192
[4,3,3] 384
[3,4,3]:
Symbol Order
[3+,4,3+] 288.1
[3,4,3]+ 576.2
[3+,4,3] 576.1
[[3+,4,3+]] 576
[3,4,3] 1152.1
[[3,4,3]]+ 1152
[[3,4,3]+] 1152
[[3,4,3]] 2304
[5,3,3]:
Symbol Order
[5,3,3]+ 7200
[5,3,3] 14400

Space groups

Rank four groups also defined the 3-dimensional space groups:

Orthorhombic

[∞,2,∞,2,∞]
Symbol
[∞,2,∞,2,∞]
[∞,2,∞,2,∞]+
[∞+,2,∞,2,∞]
[∞+,2,∞+,2,∞]
[∞+,2,∞+,2,∞+]
[∞,2,∞,2+,∞]
[∞,2+,∞,2+,∞]
[(∞,2,∞)+,2,∞]
[(∞,2,∞)+,2,∞+]
Trigonal & hexagonal
Group Symbol
[3,6,2,∞]
 [6,3,2,∞]
[6,3,2,∞]+
[6,3+,2,∞]
[(6,3)+,2,∞]
[6,3,2,∞+]
[6,3+,2,∞+]
[(6,3)+,2,∞+]
[1+,6,3,2,∞]
[1+,6,3,2,∞]+
[1+,6,3,2,∞+]
[(1+,6,3)+,2,∞]
[(1+,6,3)+,2,∞+]
[3[3],2,∞]
 [3[3],2,∞]
[3[3],2,∞]+
[3[3],2,∞+]
[(3[3])+,2,∞]
[(3[3])+,2,∞+]

Tetragonal

[4,4,2,∞]
[4,4,2,∞]
[4,4,2,∞]+
[(4,4)+,2,∞]
[4,4,2+,∞]
[4,4,2,∞+]
[(4,4)+,2+,∞]
[(4,4)+,2,∞+]
[4,4,2+,∞+]
[(4,4)+,2+,∞+]
[4+,4+,2+,∞]
[4,4+,2,∞]
[4,4+,2+,∞]
[4,4+,2,∞+]
[4,4+,2+,∞+]
Cubic
Group Coxeter Space group Index
[4,3,4]
 [4,3,4] (221) Pm3m 1
[4,3,4]+ (222) Pn3n 2
[4,3+,4] (223) Pm3n 2
[4,(3,4)+] (224) Pn3m 2
[4,3,4,1+] (225) Fm3m 2
[4,(3,4,1+)+] (226) Fm3c 4
[1+,4,3,4,1+] (227) Fd3m 4
[4,3,4,1+]+ (228) Fd3c 4
[[4,3,4]] [[4,3,4]] (229) Im3m
[[4,3,4]]+ (230) Ia3d
[[4,3+,4]]
[[4,3,4]]+
[4,31,1]
 [4,31,1] = [4,3,4,1+] 2
[4,(31,1)+] = [4,(3,4,1+)+] 4
[1+,4,31,1] = [1+,4,3,4,1+] 4
[4,31,1]+ = [4,3,4,1+]+ 4
[1+,4,31,1]+ = [1+,4,3,4,1+]+ 2
<[4,31,1]> = [4,3,4] 1
[(3,3,3,3)]
 [(3,3,3,3)] = [1+,4,31,1] 4
[(3,3,3,3)]+ = [1+,4,31,1]+ 2
<[(3,3,3,3)]> = [4,31,1] 2
[4[(3,3,3,3)]] = [4,3,4] 1

Line groups

Rank four groups also defined the 3-dimensional line groups:

Semiaffine (3D)
Point group Line group
Hermann-Mauguin Schönflies Hermann-Mauguin Offset type Wallpaper Coxeter
[∞h,2,pv]
Even n Odd n Even n Odd n IUC Orbifold Diagram
n Cn Pnq Helical: q p1 o [∞+,2,n+]
2n n S2n P2n Pn None p11g, pg(h) xx [(∞,2)+,2n+]
n/m 2n Cnh Pn/m P2n None p11m, pm(h) ** [∞+,2,n]
2n/m C2nh P2nn/m Zigzag c11m, cm(h) *x [∞+,2+,2n]
nmm nm Cnv Pnmm Pnm None p1m1, pm(v) ** [∞,2,n+]
Pncc Pnc Planar reflection p1g1, pg(v) xx [∞+,(2,n)+]
2nmm C2nv P2nnmc Zigzag c1m1, cm(v) *x [∞,2+,2n+]
n22 n2 Dn Pnq22 Pnq2 Helical: q p2 2222 [∞,2,n]+
2n2m nm Dnd P2n2m Pnm None p2mg, pmg(h) 22* [(∞,2)+,2n]
P2n2c Pnc Planar reflection p2gg, pgg 22x [∞+,2+,2n+]
n/mmm 2n2m Dnh Pn/mmm P2n2m None p2mm, pmm *2222 [∞,2,n]
Pn/mcc P2n2c Planar reflection p2mg, pmg(v) 22* [∞,(2,n)+]
2n/mmm D2nh P2nn/mcm Zigzag c2mm, cmm 2*22 [∞,2+,2n]

Wallpaper groups

Rank four groups also defined some of the 2-dimensional wallpaper groups:

Affine (2D plane)
IUC (Orbifold) Geo Coxeter
p1 (o) p1 [∞+,2,∞+]
p2 (2222) p2 [∞,2,∞]+
c2mm (2*22) c2 [∞,2+,∞]
p11g (xx) pg1 h: [∞+,(2,∞)+]
p1g1 (xx) pg1 v: [(∞,2)+,∞+]
p2gm (22*) pg2 h: [(∞,2)+,∞]
p2mg (22*) pg2 v: [∞,(2,∞)+]
IUC (Orbifold) Geo Coxeter
p11m (**) p1 h: [∞+,2,∞]
p1m1 (**) p1 v: [∞,2,∞+]
p2mm (*2222) p2 [∞,2,∞]
c11m (*x) c1 h: [∞+,2+,∞]
c1m1 (*x) c1 v: [∞,2+,∞+]
p2gg (22x) pg2g [∞+,2+,∞+]
c2mm (2*22) c2 [∞,2+,∞]

Notes

  1. ^ The Crystallographic Space groups in Geometric algebra, D. Hestenes and J. Holt, Journal of Mathematical Physics. 48, 023514 (2007) (22 pages) PDF [1]

References